| swiss-algebra-help.com |
 |
|||||||||||||||||||||
Decimals
And digits after the point (or comma). They are so called because we use the "base ten" or "decimal notation" or "denary system" when we count today. This evidence is so obvious for us that we don't remember that in other times this system did not exist. For instance, the Romans did not use them but, well, yes just the "Roman" non-positional, addition-numberal system which expressed digits with letters (I = 1, II=2, III=3, IV=4, V=5, VI=6, VII=7, VIII=8, IX=9, X=10, XX=20, XXX=30, XXXX=40, L=50, C=100, M=1000) The Roman system was not primitive or complicated as some say. Actually it's easier to calculate small numbers like they did! And the Roman numerals were used everywhere in the Roman Empire (which streched from the Adrian wall to Egypt, from North Africa to Romania) and so well used that at first the "new" decimal positional system was despised and objected when introduced in Europe. It took more than 200 year to be accepted by mathematicians! This happened during the Renaissance, around the XV century (with the help of the Gutenberg's invention: the print - 1450). But merchants, kept using the roman numerals for another 100 years, though! Decimals are an invention of such an incredible importance that the person who developed it (not invented) could not and did not realize its wideranging effects fully. [1] It opened a new frontier within the mathematics universe which is still unfathom today [2]: the evidence that more numbers exist than we originally thought of. The person was an Belgian engineer who lived in the XVI-XVII century: Simon Stevin (1548-1620). It all started by the "discovery" of the decimal notation in Europe in the late middleages, by an Italian merchant travelling in the South Mediterranean with his son Leonardo Pisano, in the XII century (but "mathematical" contacts with the two cultures, the European and the "arab" one were much earlier, since the IX century). Romans' Calculation Let's calculate 11+2 XI + II = XIII
Now let's do 26 + 12 or XXVI + XII
Anyhow, properly said, a decimal is a number which occur after "a point" and take a place value according to their position: 0.1 = 1 tenth 0.01 = 1 hundreth 0.001 = 1 thousandth exactly like the natural numbers but in reverse order: 10= 1 ten 100 = 1 hundred 1000 = 1 thousand As for the definition, here it is from the book The A to Z of Mathematics: A Basic Guide
So for instance 3/10 = 0.3 3/100= 0.03 3/1000= 0.003 and so And what about those fractions which have NOT a denominator as power of 10? Than you have to convert them ,in this way... Easy? Not at all! You are just beginning to understand rational numbers. Not sure what I am talikg about? Let's then explore those strange numbers that you sometimes see on you calculator, like 1,6666666......and never ending. They are called repeating decimals and they have a surprise for you! Check it out! By the way...any calculator of roman numerals? Here it is: Numbers To Roman Numerals Converter=
Source:
From decimals to the algebra homework help webpage
|
On the shore of a vast sea
|
|||||||||||||||||||||
|
Stay in touch with nature. It's full of mathematics! 
Enjoy This Site?
Then why not use the button below, to add us to your favorite bookmarking service? |
||||||||||||||||||||||
|
| Homepage| About Us |Study Tips |Contact | This Website is Powered by Site Build It! - and I would never use anything else.
|
||||||||||||||||||||||
|
| ||||||||||||||||||||||
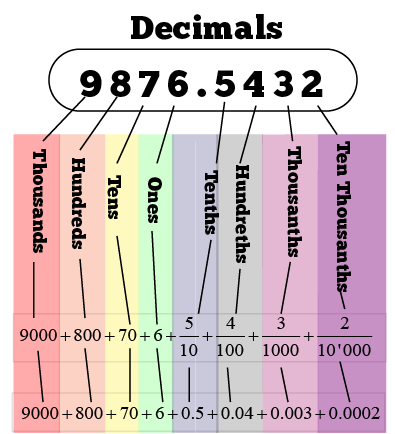 Decimals are those numbers which have a point (or a comma).
Decimals are those numbers which have a point (or a comma).